Fraction Exponent Calculator
Fraction Exponent Calculator is a free online tool that helps you quickly solve fractional exponents of the form $x^{\frac{n}{d}}$. It also provides detailed, step-by-step explanations, making it perfect for learning, practicing, and quick reference—all available on CalculationClub.com.
Simply enter a base number along with the numerator and denominator of the exponent, and the Fraction Exponent Calculator will instantly compute the result. For example, inputting base = 8, numerator = 2, denominator = 3 will calculate $8^{\frac{2}{3}}$ and show how to simplify it step-by-step.
In addition to the final result, the calculator provides a clear explanation of each step, including how to handle roots and powers. You can also toggle the visibility of detailed steps based on whether you want a quick result or a deeper understanding.
How to Use the Online Fraction Exponent Calculator?
1. Enter the Base – In the field labeled X (base), type the base number you want to raise to a fractional exponent. For example, enter 8 if you want to calculate $8^{\frac{2}{3}}$.
2. Enter the Numerator – In the field labeled n (numerator), enter the numerator of the fractional exponent. For example, enter 2 for $8^{\frac{2}{3}}$.3. Enter the Denominator – In the field labeled d (denominator), enter the denominator of the fractional exponent. For example, enter 3 for $8^{\frac{2}{3}}$.
4. Click “Calculate” – Press the Calculate button to compute the value of the fractional exponent.5. View the Result – The calculator will instantly display the result of your expression. For example, entering base = 8, numerator = 2, denominator = 3 will show that $8^{\frac{2}{3}} = 4$.
6. Show or Hide Steps – Click Hide Steps to toggle the visibility of the detailed, step-by-step explanation showing how the fractional exponent was evaluated (e.g., converting the denominator into a root, then applying the power).
7. Reset for a New Calculation – Click Reset to clear all input fields and start a new calculation.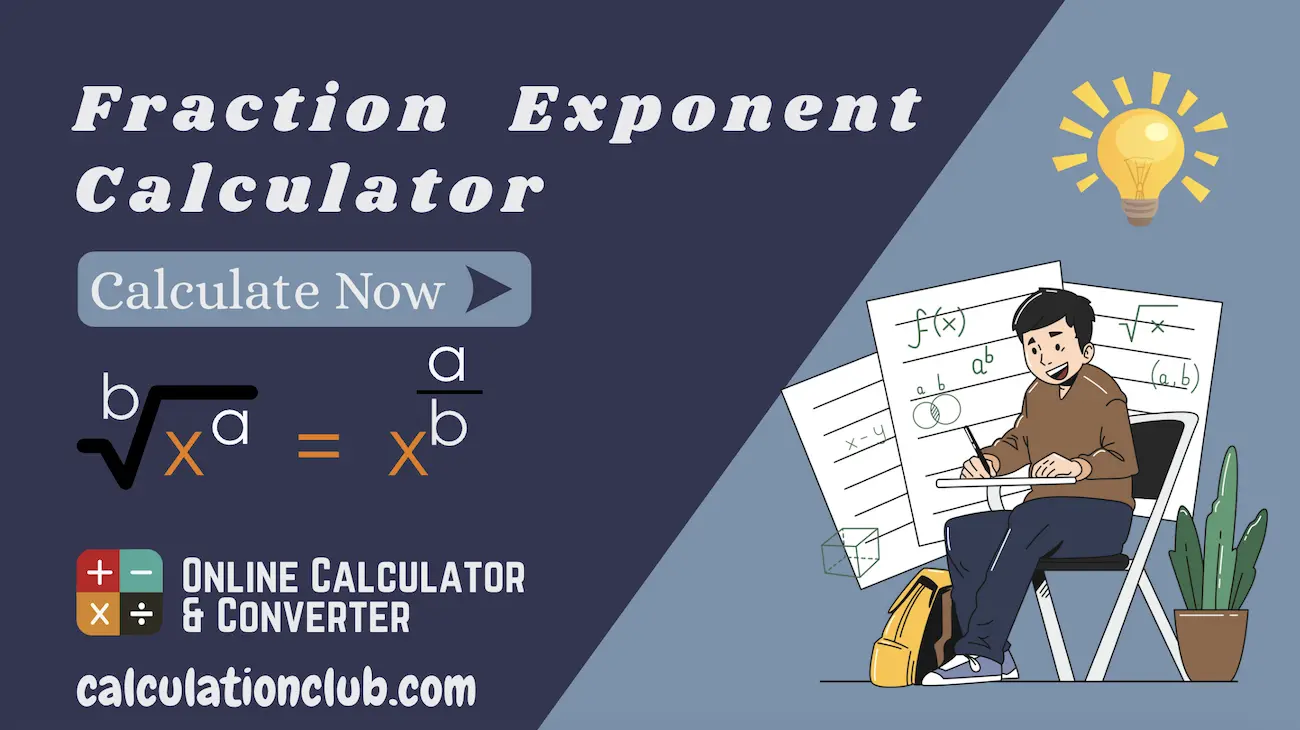
Fraction Exponent Calculator
What is a Fraction Exponent Calculator?
A Fraction Exponent Calculator is a powerful and user-friendly online tool that helps you calculate the result of raising a base number to a fractional exponent. Whether you’re entering an expression like $8^{\frac{2}{3}}$ or $27^{\frac{4}{3}}$, this calculator quickly provides the result along with step-by-step explanations.
What are Fractional Exponents?
Fractional exponents represent both roots and powers combined into a single expression.
In simpler terms: An expression like $x^{\frac{n}{d}}$ means you take the $d$-th root of $x$ and then raise it to the $n$-th power.
Or equivalently: $x^{\frac{n}{d}} = \left( \sqrt[d]{x} \right)^n = \sqrt[d]{x^n}$where:- $x$ is the base,
- $n$ is the numerator (power),
- $d$ is the denominator (root).
How to Manually Calculate Fractional Exponents
To manually calculate fractional exponents, follow these steps:
1. Understand the Expression
Recognize that an expression like $x^{\frac{n}{d}}$ means you are both taking a root and applying a power.2. Find the Root First
Take the $d$-th root of the base $x$.This means calculating $\sqrt[d]{x}$.
3. Apply the Power
After finding the root, raise the result to the $n$-th power.
That is, compute $\left( \sqrt[d]{x} \right)^n$.Example 1 (Positive Exponent):
- Step 1: Find the fourth root of 16: $\sqrt[4]{16} = 2$
- Step 2: Raise it to the power of 3: $2^3 = 8$
$16^{\frac{3}{4}} = 8$
Example 2 (Positive Exponent):
Calculate $81^{\frac{1}{2}}$- Step 1: Find the square root of 81: $\sqrt{81} = 9$
Answer:
$81^{\frac{1}{2}} = 9$Example 3 (Negative Exponent):
Calculate $8^{-\frac{2}{3}}$- Step 1: Find the cube root of 8: $\sqrt[3]{8} = 2$
- Step 2: Raise it to the power of 2: $2^2 = 4$
- Step 3: Apply the negative exponent: $\frac{1}{4}$
Answer:
$8^{-\frac{2}{3}} = \frac{1}{4}$Example 4 (Negative Exponent):
Calculate $27^{-\frac{1}{3}}$- Step 1: Find the cube root of 27: $\sqrt[3]{27} = 3$
- Step 2: Apply the negative exponent: $\frac{1}{3}$
$27^{-\frac{1}{3}} = \frac{1}{3}$
FAQs on Fraction Exponent Calculator?
Q1: What is a fraction exponent?Ans: A fraction exponent represents a combination of a root and a power. The numerator of the fraction is the exponent applied to the base, and the denominator is the root of the base. For example, $a^{\frac{m}{n}}$ means the nth root of $a$, raised to the power of $m$.
Q2: How do I calculate a fraction exponent?
Ans: To calculate a fraction exponent, first take the root corresponding to the denominator of the fraction, then raise the result to the power of the numerator. For example, $8^{\frac{2}{3}}$ means finding the cube root of 8, which is 2, and then squaring it: $2^2 = 4$.
Q3: Can a fraction exponent result in a negative number?
Ans: Yes, fraction exponents can result in negative numbers, especially when the base is negative. For instance, $(-8)^{\frac{2}{3}}$ will first find the cube root of -8, which is -2, and then square it, yielding 4.Q4: What happens if the denominator of the fraction exponent is 1?
Ans: If the denominator of the fraction exponent is 1, the calculation reduces to a regular power. For example, $9^{\frac{3}{1}} = 9^3 = 729$. Essentially, it is just raising the base to the power of the numerator.
Q5: How do I calculate negative fraction exponents?
Ans: Negative fraction exponents follow the same rules as positive exponents but with an added step: you take the reciprocal of the base and then apply the fraction exponent. For example, $8^{-\frac{2}{3}}$ means first calculating $8^{\frac{2}{3}}$ and then taking the reciprocal of the result: $\frac{1}{4}$.
Conclusion: The Fraction Exponent Calculator is a simple yet powerful tool for calculating fractional exponents. It helps you quickly solve problems involving roots and powers, providing accurate results and clear step-by-step explanations. Perfect for students and anyone working with exponents, it simplifies complex calculations with ease.
My Request to All: If you enjoy using my Fraction Exponent Calculator and my website, please consider sharing the link to this page or the website with your friends. Additionally, if you have any requests, complaints, suggestions, or feedback, feel free to reach out via our WhatsApp channel or Telegram group.
Telegram Link – Join CalculationClub on Telegram
YouTube Link – Watch CalculationClub on YouTube
For more tools, please visit our homepage at Calculationclub
For additional tools in Hindi, you can visit Meter to Feet
Thank you for your support!